每日一题[9] “奔驰定理”与五心的向量表达 |
您所在的位置:网站首页 › 垂心向量表示证明 › 每日一题[9] “奔驰定理”与五心的向量表达 |
每日一题[9] “奔驰定理”与五心的向量表达
|
下面的这个习题可以称得上是平面向量中最优美的一个结论,并且这个结论对于利用平面向量解决平面几何问题,尤其是解决跟三角形的五心相关的问题,有着决定性的基石作用.
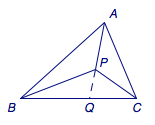
延长\(AP\)交边\(BC\)于点\(Q\),则
\[\overrightarrow{AP}=\dfrac{\triangle APB+\triangle CPA}{\triangle ABC}\overrightarrow{AQ},\] 且根据共线向量的表达,有 \[\overrightarrow{AQ}=\dfrac{\triangle APB}{\triangle APB+\triangle CPA}\overrightarrow{AC}+\dfrac{\triangle CPA}{\triangle APB+\triangle CPA}\overrightarrow{AB}.\] 从而可得 \[\overrightarrow{AP}=\dfrac{\triangle APB}{\triangle ABC}\overrightarrow{AC}+\dfrac{\triangle CPA}{\triangle ABC}\overrightarrow{AB}.\] 对上式应用向量的换底公式(\(\overrightarrow{AB}=\overrightarrow{MB}-\overrightarrow{MA}\)),将所有的向量改写为以\(P\)为起点的, \[-\overrightarrow{PA}=\dfrac{\triangle APB}{\triangle ABC}\left(\overrightarrow{PC}-\overrightarrow{PA}\right)+\dfrac{\triangle CPA}{\triangle ABC}\left(\overrightarrow{PB}-\overrightarrow{PA}\right),\] 整理即得. 由于这个定理和奔驰的logo很相似,我们把它称为奔驰定理.
这个定理也可以利用三角恒等式证明,记\(\angle APB=\alpha\),\(\angle APC =\beta\)、\(PA=x\)、\(PB=y\)、\(PC=z\),欲证等式左边与\(\overrightarrow{PA}\)作数量积\[\begin{split}&\quad \left(s_A\overrightarrow{PA}+s_B\overrightarrow{PB}+s_C\overrightarrow{PC}\right)\cdot\overrightarrow{PA}\\&=\dfrac 12yz\sin\left[2\pi-\left(\alpha+\beta\right)\right]\cdot x^2+\dfrac 12zx\sin\beta\cdot xy\cos\alpha+\dfrac 12xy\sin\alpha\cdot zx\cos\beta\\&=\dfrac 12x^2yz\left[-\sin\left(\alpha+\beta\right)+\cos\alpha\sin\beta+\sin\alpha\cos\beta\right]\\&=0,\end{split}\]同理,欲证不等式左边与\(\overrightarrow{PB}\)、\(\overrightarrow{PC}\)作数量积得到的结果也均为\(0\).而向量\(\overrightarrow{PA}\)、\(\overrightarrow{PB}\)、\(\overrightarrow{PC}\)不共线,因此欲证明等式左边为零向量,等式得证. 事实上,根据这个定理,我们容易得到三角形的五心的向量表达: 重心\(G\)满足 \[\overrightarrow{GA}+\overrightarrow{GB}+\overrightarrow{GC}=\overrightarrow 0,\] 外心\(O\)满足 \[\sin 2A\overrightarrow{OA}+\sin 2B\overrightarrow{OB}+\sin 2C\overrightarrow{OC}=\overrightarrow 0,\] 内心\(I\)满足 \[\begin{split}a\overrightarrow{IA}+b\overrightarrow{IB}+c\overrightarrow{IC}=\overrightarrow 0,\\\sin A\overrightarrow{IA}+\sin B\overrightarrow{IB}+\sin C\overrightarrow{IC}=\overrightarrow 0\end{split}\] 垂心\(H\)满足 \[\tan A\overrightarrow{HA}+\tan B\overrightarrow{HB}+\tan C\overrightarrow{HC}=\overrightarrow 0,\] 旁心\(I_A\)满足 \[-\sin A\overrightarrow{I_AA}+\sin B\overrightarrow{I_AB}+\sin C\overrightarrow{I_AC}=\overrightarrow 0.\] 三角形的五心的向量表达是用向量法解平面几何问题的重要理论基础. |
【本文地址】
今日新闻 |
推荐新闻 |
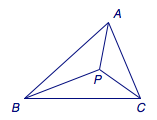
 已知\(P\)为三角形\(ABC\)内一点,求证:\[s_A\overrightarrow{PA}+s_B\overrightarrow{PB}+s_C\overrightarrow{PC}=\overrightarrow 0,\]其中\(s_A\)、\(s_B\)、\(s_C\)分别是\(\triangle BPC\)、\(\triangle CPA\)、\(\triangle APB\)的面积.
已知\(P\)为三角形\(ABC\)内一点,求证:\[s_A\overrightarrow{PA}+s_B\overrightarrow{PB}+s_C\overrightarrow{PC}=\overrightarrow 0,\]其中\(s_A\)、\(s_B\)、\(s_C\)分别是\(\triangle BPC\)、\(\triangle CPA\)、\(\triangle APB\)的面积.